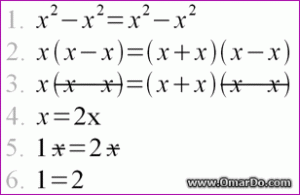Christmas Maths Madness

I was meant to do this on Monday, but it was Christmas Eve and I was busy doing, well, Christmas-y things... So here goes.

On the fourth day of Christmas, Mashematics gave to me...

... four Christmas Maths pictures! Merry Christmas :)

Monday Maths Madness 21

I have more jokes this week!

Never name your son pi. Pi's a funny name isn't it? It's like if someone goes up to you and asks what your name is, you can be like 'Pi'. But then they'd say 'no, what's your full name?' You'd be all like 'Don't ask man, we'd be here forever


Why did the chicken cross the Mobius strip?

To get to the other... wait...


Your Mum's so mean, she has no standard deviation!


Two cats are sliding down a roof at the same time - which one falls off first?

The one with the smaller mu



Sorry for the pathetic amount this week. I've been busy today, and I'm about to play Lego Batman 2 with my sister for the first time in 10 weeks, so I'm a little distracted!

Monday Maths Madness 20 - Answers

As promised, here are the answers to Monday's puzzles.

1. Christmas Past, Christmas presents

Answer: On the 12th day alone, my true loves sends to me 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 + 9 + 10 + 11 + 12 = 78 presents.

Over the duration of the 12 days, my true love sends to me 12 partridges in a pear tree, 11 lots of 2 turtle doves, 10 lots of 3 French hens, and so on. In other words (or numbers I suppose) I get 2 x ((1 x 12) + (2 x 11) + (3 x 10) + (4 x 9) + (5 x 8) + (6 x 7)) = 364 presents. Which is one for every day of the year except Christmas!

2. What to wear where

We know that Miss Black can't be wearing the black dress, but she also can't be wearing green, as the woman in green answered her. Therefore, Miss Black is wearing white, Miss Green is wearing black and Miss White is wearing green.

3. A toast to toast

First, toast one side of slices 1 and 2. Second, toast the other side of slice 1, and the first side of slice 3. Third, toast the second side of slice 2 and 3. Then you have toasted both sides of 3 slices in just three toastings.

Well done if you got the answers :)

Monday Maths Madness 20

Today is the last Monday of my first term at university. It has gone by so quickly, but I am ready to come home at the weekend. I keep counting down the days, I can't wait! In the meantime, I have run out of pictures, so I think it's time for more puzzles. These are from Ball of Confusion again.


As I am sat here learning Christmas songs for Glee club, I feel it is appropriate to start with a Christmas puzzle.

Christmas past, Christmas presents

On the first day of Christmas my true love sent to me, a partridge in a pear tree. That was 1 present, but on the second day of Christmas, my true love sent to me, 2 turtle doves and a partridge in a pear tree. That was 3 more presents - 4 in total. We know, of course, that this continued for the entire 12 days of Christmas.

How many presents arrived on the 12th day of Christmas? How many presents arrived over the entire 12 days?

What to wear where

Three girls turned up to a party in a black dress, a green dress and a white dress. They were Miss Black, Miss Green and Miss White. Miss Black said, "It's amazing - our dresses match our names, but none of us is actually wearing the dress that matches her name."

The girl in green said "So what?".

Who was wearing which dress?

A toast to toast

You only have a grill and space for two pieces of bread under it, so it takes 4 toastings to do 3 slices on both sides. Or does it?

Can you toast 3 pieces of bread on both sides in less than 4 toastings?

That's all from me this week. I would find more, but I have songs to practise. Answers on Wednesday!

Monday Maths Madness 19

Monday again. So I still haven't done the post about irrational numbers that I've been planning to do for like, the last month. But! I did at least do a different post. So I'm not completely hopeless. Anyway...

Yep, this basically sums up (no pun intended) maths at Warwick University! It's not all bad. It's just kinda hard. Strangely enough I enjoy it though.

Ok, you can't really see that picture very well... But basically it's more maths love. Big fan of the maths love me.

EDIT: Ok, the last picture I put up I'd already used a couple of weeks ago. Note to self, update my photo folder. So I'll use this one instead! I don't really follow politics, like, at all, so I can't say for sure if this is true... But I imagine it is.

One day, maybe, I will write my blog post on irrational numbers. But I'll probably just be saying the same thing next Monday... so see you then!

A is for All Nighter

A while ago, my mum and my sister both started going on alphabet dates. Basically, each date is something to do with each letter of the alphabet, starting from A and eventually ending with Z. Now, since coming to University, I haven't been able to see much of my boyfriend. I went from seeing him every day to a couple of days every 2 weeks or so. This meant that we've been trying to come up with a plan to do something nice when we meet up, rather than just slobbing around like we normally do.

Last weekend, the student cinema put on an Allnighter, and we decided to go. But I was thinking about it one night, and realised that Allnighter begins with an A. This got me thinking about the alphabet dates, and how that might be a good way for us to spend our time together. It would give us more to get excited about on our visits. I told my boyfriend, and he immediately started thinking of things we could do for other letters, so I think that we are definitely going through with it!

A is for All-Nighter

Neither of us had ever been to an all-nighter before, so we were both pretty excited. After stocking up on loads of chocolate, sweets, fizzy drinks and biscuits to keep us awake, we headed down to the lecture theatre where the student cinema is held. We were given a running order on what was going to happen during the night, and then the first film started.

Ted - Ted is a film about a little boy called John, who wishes is teddy was real so he would have a friend. His wish comes true and the two grow up together. Years later, John and Ted are still best friends, but their friendship is tested when John has to choose between the vulgar teddy bear, or his girlfriend of 4 years.

I didn't think I was going to like Ted. It's done by the same guy who does Family Guy, which I cannot stand, but I actually quite enjoyed it. It was completely ridiculous and stupid, but there were some moments that I couldn't help laughing at. Mostly the bit at the end when they said the fat kid got a personal trainer and became Taylor Lautner... You probably have to see it to find that funny... Anyway, it was a good start to the evening.

Brave - Not wanting to be forced into marriage, the Scottish princess Merida falls out with her mother, the Queen, and finds a witch to cast a spell to make her change her mind. The spell goes horribly wrong (don't they always?) and Merida has to repair the bond that was broken before her mother is cursed to be a bear forever.

I had no idea what Brave was actually about. Disney has a way of making trailers entirely out of clips that aren't included in the movie, but I knew I really wanted to see it. And it didn't disappoint! I'll admit the strong Scottish accents made it a little hard to understand what they were saying sometimes, but it was funny, sad and heartwarming all at the same time. Also, the baby bears were the cutest damn things I've ever seen in my life.

Usual Suspects - (Summary from IMDB) A boat has been destroyed, criminals are dead, and the key to this mystery lies with the only survivor and his twisted, convoluted story beginning with five career crooks in a seemingly random police lineup.

I did watch this film, but I'm not sure I could really explain what happened. It was getting pretty late, and there was a lot of skipping between flashbacks and current events, I got kind of confused. I did enjoy it though, and would watch it again, maybe earlier in the day, so I could understand what goes on.

Mystery Film - This is where the night started to go wrong really. Up until this point we were having a really good time watching films, losing quizzes and not winning any prizes in the raffle (I know that sounds bad, but we were having fun!) Then it got to the mystery film. Before the night, we were told what 5 out of the 6 films were, leaving one film as a surprise for the night. I was quite excited to find out what it would be, but the reality was disappointing. The film was This is Spinal Tap. I'd never heard of it, but within 10 minutes I was bored and decided to have a nap. The boyfriend was moaning about how rubbish it was every time I woke up, but for some reason thought it would be a good idea to stay up and watch it anyway. So yeah, mystery film, not so good.

Bourne Legacy - I also slept through this one... I would have watched it if it was on earlier, but it was close to 4 in the morning, and I couldn't keep my eyes open. So I have no idea if this was any good or not.

Jaws - I love Jaws, and I woke up from my two-movie nap specifically so I could watch it. Unfortunately my boyfriend felt ill after too many sugary drinks and nearly 24 hours of being awake on top of the hour and a half drive to get here, so at this point (at 6.30 in the morning!) we decided to call it quits. To be fair, he had watched all five movies up to that point. I watched 3, and slept through 2, so he did more of an all nighter than me!

So that was it. It was kind of weird leaving the cinema in daylight when it was dark when we arrived. It was also weird getting ready for bed at nearly 7am, but we needed the sleep. We did spend the rest of the weekend slobbing around watching Criminal Minds, but I think we deserved the rest!

Being the brilliant photographer I am, I only have one photo of the entire night...

Highlight of the date: Brave was my favourite film of the night I think. Disney for the win!
Lowlight of the date: When I forgot to bring my ticket with me when I went for a toilet break. I think the guy only let me back in because I wasn't wearing any shoes...

Monday Maths 18

I know I promised a while ago that I would be writing another blog post, but I've been so busy I've just not gotten round to it. This week I promise. Seriously this time!

This is how I feel pretty much all of the time. Actually, I lie. Most of the time I have no idea what I'm doing. But when I think it's too easy it doesn't seem right!

I'm not really sure what to say about this one, other than to agree that it is a little sad.

This last one is an alternative solution to the popular Wolf, Goat, Cabbage problem. Although, I have to say I would keep the wolf over the goat. Wolves are cool.

Monday Maths Madness 17

It's Monday again! I've had a mad day running from lecture room to lecture room giving serenades with Glee club while students and lecturers have been getting lap dances from people in dolphin, turtle and gorilla costumes. No lie. RAG week, it's crazy. Anyhoo, time for a different kind of madness now. Maths madness!

I think it is one of the best feelings in the world when you understand a problem, and suddenly everything makes perfect sense. I've had a few of those moments this term. They felt good.

I really want this clock. That is really nerdy isn't it?

I'm sure some of my lecturers are close to doing this sometimes...

That's it from me today. I need some rest for more RAG craziness tomorrow.

Monday Maths 16

I have more pictures for you today :)

My boyfriend really liked this one. I think it's mostly because he actually got it, but I think it's funny too. I've been doing a lot about converging sequences in Analysis recently.

Why is 6 scared of 7...?

I told you that Fibonacci pops up in nature all the time! Even in the shape of storms.

I realise that I really ought to write an actual blog post sometime soon. I have an idea for one, but it's finding the time/energy/words to write it that is slowing me down. Soon!

Monday Maths Madness 15

As it is Hallowe'en this Wednesday, I have some Hallowe'en themed maths madness! Muahahaha

This is probably the only kind of pumpkin pie I like...

More pumpkin pi! Apparently there aren't a lot of Hallowe'en maths jokes on the internet. In fact I could only find one other. This one seems really popular out on the web.

Why do mathematicians sometimes confuse Halloween and Christmas?
Because Oct 31 = Dec 25.

Basically counting to 31 using base 8 is the same as counting to 25 using base 10 (and yes I did just have to write out all the numbers to check I was explaining the joke right...). It just so happens that October 31st and December 25th are significant dates in the calendar. Spooky!

Happy Hallowe'en everyone! :)

The Dominoes and the Chessboard

On Monday, I posted a puzzle regarding a chessboard and some dominoes. As promised, I am now going to give the answer (so that my boyfriend's family will stop arguing about it!)

So, is it possible to place 32 dominoes, each covering two squares, on a chessboard with two diagonally opposite corners cut out?

The answer is: No. It is not possible.

But how do you prove it? Most of the modules in my degree this term are all about proving things, and it can get very confusing. Especially Analysis. But there is a simple way to think about this puzzle.

As the two corners cut out of the chessboard are diagonally opposite each other, they must be the same colour. This means that we have 30 of one colour (in the picture above it is black) and 32 of the other colour (obviously, in this case white). As the dominoes only cover two squares, no matter how you place them, they are going to cover one of each colour. So if we pair off the colours, we can match 30 black to 30 white, but then we are left with 2 of the same colour left over. We know that a single domino cannot cover two squares of the same colour, so the puzzle is therefore impossible.

Hopefully I explained that clearly so you aren't left feeling as confused as before!

Monday Maths Madness 14

This week I have another puzzle for you to ponder over (just one this time, I promise!) I think I've heard of this puzzle before, but I read about it recently in Mathematics: A Very Short Introduction by Timothy Gowers and thought I would share.

The basic idea, is you have a chess board with two corners cut out like so:

 

You also have 31 dominoes that each cover two squares. Can you lie all of the dominoes on the chessboard, so they cover it completely?

Answer in a few days!

Edit: Ok, it's not totally obvious from the picture, but the top left and bottom right squares have been cut out.

Monday Maths Madness 13

Back to pictures this week. My boyfriend keeps sending me links to pictures on 9gag.com that are maths related, so I have enough to do a post about them!

This is a bit what I feel like in my lectures at the moment! Especially Analysis. You think it's simple, but when you actually come to write it down, there are no words to explain it.

The square root of a negative number is called an imaginary number. Anything else is a real number. Squaring an imaginary number makes it real. Shit just got real.

This is my personal favourite. I'm doing a lot about planes in Intro to Geometry at the moment. So far there have been no snakes though... Just triangles.

A nice surprise

I like getting maths book shaped presents :) Thank you Kirk!

Monday Maths Madness 12 (part 2)

As promised, here are the answers to the puzzles I gave on Monday.

1. Fumbling in the dark.

I would need to take 4 shoes to be sure of having a pair that match. If you took only 3 shoes, you could have taken one of each pair, so taking one more ensures you definitely have a pair of matching shoes.

Similarly, I would need to take 3 socks to be sure of having a pair that match.

2. Animal Farm

He would still have 50 horses - calling a cow a horse doesn't make it a horse.

3. Sum for simpletons

56 = 7 x 8

4. An up and down kind of existence

There is an absolute certainty that the monk will be at the same point on the road at the same time of day. Because the monk leaves later and arrives earlier on the second day than on the first day, there isn't a time of day on the second day that he was walking when he wouldn't have been on the first day. Therefore, if you imagine both journeys being made on the same day by two different monks, there comes a point where the two monks would have to walk past each other.

Monday Maths Madness 12

For anyone who is into Strictly Come Dancing, you will know that one of the celebrities is Johnny Ball. Johnny Ball is a TV personality from the 70s and 80s, and is known for popularising maths and science for children.

A little while ago, I can't remember exactly when, my mum bought me a book written by Johnny Ball. It is called Ball of Confusion, which is a collection of puzzles to do with maths, inspired by when he presented a puzzle on Zoe Ball's (his daughter) Radio 2 show. As Strictly just started this last weekend, I thought I would give a few puzzles from the book for you to ponder over.

1. Fumbling in the Dark

The lights have gone out - I said we should never trust wind farms - and I have to search my wardrobe in the dark for a pair of shoes and socks.

I have 3 pairs of shoes, 12 pairs of black and 12 pairs of brown socks.

How many of each do I need to take, to be sure I have a matching pair of each to wear?

2. Animal Farm

A farmer has 20 goats, 30 cows and 50 horses. How many horses would he have if you called the cows 'horses'?

3. Sum for simpletons

Look at this: 12 = 3 x 4.

Can you think of four other consecutive digits that do that?

4. An up and down kind of existence

A monk lives on a river bank in a valley. Once a week he must travel to the monastery at the top of the Lonely Mountain. He sets off at dawn, as the road is steep and long, and he arrives as the Sun sets. Next morning he has a lie in and leaves for home at 10am. As the road is downhill all the way, he arrives home at around 3pm.

What are the chances of the monk being at exactly the same point on the road, at exactly the same time on both days?

Answers on Wednesday! (Just to annoy my sister :P)

Monday Maths Madness 11

I have a few A Mathematician And... jokes for you this week.

A physicist and a mathematician are sitting in a faculty lounge. Suddenly, the coffee machine catches on fire. The physicist grabs a bucket and leap towards the sink, filled the bucket with water and puts out the fire. Second day, the same two sit in the same lounge. Again, the coffee machine catches on fire. This time, the mathematician stands up, got a bucket, hands the bucket to the physicist, thus reducing the problem to a previously solved one.

A biologist, a physicist and a mathematician were sitting in a street cafe watching the crowd. Across the street they saw a man and a woman entering a building. Ten minutes they reappeared together with a third person.
- They have multiplied, said the biologist.
- Oh no, an error in measurement, the physicist sighed.
- If exactly one person enters the building now, it will be empty again, the mathematician concluded.

Several scientists were all posed the following question: "What is 2 * 2 ?"
The engineer whips out his slide rule (so it's old) and shuffles it back and forth, and finally announces "3.99".
The physicist consults his technical references, sets up the problem on his computer, and announces "it lies between 3.98 and 4.02".
The mathematician cogitates for a while, then announces: "I don't know what the answer is, but I can tell you, an answer exists!".
Philosopher smiles: "But what do you mean by 2 * 2 ?"
Logician replies: "Please define 2 * 2 more precisely." 
The sociologist: "I don't know, but is was nice talking about it".
Behavioral Ecologist: "A polygamous mating system".
Medical Student : "4" All others looking astonished : "How did you know ??" Medical Student : :I memorized it." 

A physicist, a mathematician, and a mystic were asked to name the greatest invention of all time. The physicist chose the fire, which gave humanity the power over matter. The mathematician chose the alphabet, which gave humanity power over symbols. The mystic chose the thermos bottle.
"Why a thermos bottle?" the others asked.
"Because the thermos keeps hot liquids hot in winter and cold liquids cold in summer."
"Yes -- so what?"
"Think about it." said the mystic reverently. That little bottle -- how does it *know*?"

A mathematician, a physicist, and an engineer were traveling through Scotland when they saw a black sheep through the window of the train.
"Aha," says the engineer, "I see that Scottish sheep are black."
"Hmm," says the physicist, "You mean that some Scottish sheep are black."
"No," says the mathematician, "All we know is that there is at least one sheep in Scotland, and that at least one side of that one sheep is black!"

A mathematician, a physicist, and an engineer are all given identical rubber balls and told to find the volume. They are given anything they want to measure it, and have all the time they need. The mathematician pulls out a measuring tape and records the circumference. He then divides by two times pi to get the radius, cubes that, multiplies by pi again, and then multiplies by four-thirds and thereby calculates the volume. The physicist gets a bucket of water, places 1.00000 gallons of water in the bucket, drops in the ball, and measures the displacement to six significant figures. And the engineer? He writes down the serial number of the ball, and looks it up.

There are many more here. Enjoy!

My New Home

Today was a big day. I moved into my room at University. I don't really feel ready for it. I don't think I've ever cried so much when my mum and my boyfriend left.

But my room is really nice. It is a decent size, and has everything I need in it to help me survive my first year at Uni.

I am going to miss everyone so much, but I still want to have a good time. Right now I still feel a bit helpless, but it should get better. I have an introductory lecture tomorrow morning, so that should clear some things up for me about my course, and I get to meet my personal tutor.

Can you tell I'm a maths student?

This is the start of a very big adventure.

Fibonacci

Leonardo da Pisa (otherwise known as Fibonacci) was an Italian mathematician born around 1770. Fibonacci is known for popularising the Arabic numerals (1, 2, 3 etc.) in Christian Europe, but is probably most famous for the number sequence he published in his book, Liber Abaci. The Fibonacci Sequence. This sequence of numbers was actually already known to Indian mathematicians as early as the 6th Century, but Fibonacci was the one who introduced it to the West.

Fibonacci originally came across the sequence when trying to solve a problem to do with the growth rate of rabbit populations. His problem was this:

Let a pair of baby rabbits into a fenced off garden. After a month they will mature into adult rabbits. Every month after that they will produce another pair of rabbits. If every pair of rabbits follows this model, and assuming none of the rabbits die, how many rabbits will there be after a year?

Obviously this theoretical model was created based on some very crude assumptions. For starters, the rabbits never die (which clearly wouldn't happen). Also it assumes that rabbits mate for life, they consistently produce another pair every month who also mate for life, and only take a month to mature into rabbits who can reproduce. These assumptions meant that he wasn't accurately predicting the growth rates of rabbits, but he did produce a sequence that is still used today, in science and in nature!

This image shows how the model plays out when you apply the limitations and run it through. The orange rabbits are the babies, and the white rabbits are when they have matured. When an arrow points from a white pair to a white pair, it shows the same pair of rabbits. The sequence Fibonacci ended up with was 1, 1, 2, 3, 5, 8, 13, 21... and so on. This is the famous Fibonacci Sequence. It is so famous because it has some interesting properties.

• The most obvious property is that every term in the sequence is the sum of the previous two. For example, 1+1=2, 1+2=3, 2+3=5 and so on.
• When you add the terms of the sequence (1+1=2, 1+1+2=4, 1+1+2+3=7) the answers create a new sequence that is similar to the original. When you add n terms of the Fibonacci Sequence together, the total equals the (n+2)th (for want of a better description...) term minus 1. For example, if you add the first 5 terms of the Fibonacci Sequence, the answer is the 7th term of the Fibonacci Sequence minus 1.

Original  1   1   2   3   5   8   13   21   34   55...
New                       2   4   7   12   20   33   54...

• When you add the squares of the Fibonacci numbers, you get another sequence. It just so happens there is an easy way to work out the sum up to the nth term of the squared sequence by using the Fibonacci Sequence. Say you want to work out the 5th term of the sum of squares, you take the 5th and 6th Fibonacci numbers and multiply them together. It comes out to the same number. In this case, 5x8=40.

Original                1   1   2   3       5     8       13       21      34        55...
Squares                1   1   4   9       25   64     169     441    1156    3025...

Sum of Squares    1   2   6   15    40    104   273    714     1870   4895

When you find the ratio between successive Fibonacci numbers, the ratios soon approach the number known as the Golden Ratio. The Golden Ratio is an irrational number (meaning the decimals don't end, and don't repeat in a pattern) that equals 1.618 to 3dp. The Golden Ratio (depicted by the Greek letter phi φ) can be shown as a rectangle, where the ratio of a:b is the same as the ratio of a+b:a (referring to the sides in the picture below).

When you draw the Fibonacci numbers as squares with side lengths corresponding to the number in the sequence, you end up with a picture like this.

Ignore the random line, I didn't count it correctly... Anyway, presenting the Fibonacci numbers in this way shows that it is a good approximation for the Golden Rectangle. The Golden Rectangle was thought to be the most pleasing rectangle by ancient scholars, and is used by many artists and architects. For example, the Parthenon in Athens, Greece, follows the Golden Rectangle, as does Leonardo da Vinci's Mona Lisa.

If you join the corners of the Golden Rectangle with a curved line, you end up with a spiral, known as (surprise, surprise) the Golden Spiral. The Fibonacci spiral is also a good approximation to this.

The Golden Spiral is found everywhere in nature. For example, the patterns on many different shells, such as snail shells, follows the same spiral. And of course, the picture that started off all of this research of mine:

That's it from me on Fibonacci. I've been trying to write this blog post for so long now, I think it's about time I actually finished it off and posted it.

Monday Maths Madness 10

This is one I see all over the place, especially on Facebook. It's pretty self explanatory I think. Simple and funny.

sin(x) doesn't quite work like that... Extra points for creativity though maybe? And they are right, six does equal 6.

This is one to hurt your head. I've not actually worked out where the contradiction actually comes into it. It's too late for that right now... (I'm very tired ok!) This is exactly the sort of thing that makes my sister hate maths. She still can't convince me though :)

Four pictures today, you lucky things! This one a friend sent to me. I didn't actually know that one Newton per square metre is a Pascal, but I think you get the jist of the joke anyway (well, I did). It made me giggle, so I thought I'd share it.

Well that's it for this week. Enjoy :)

Monday Maths Madness 9

I don't have any pictures this week, so I thought I would share some interesting (well I think so anyway!) facts about a number everyone knows: 666.

The number 666 is probably most commonly known as the Number of the Beast, from the biblical book of Revelation. However, it also has some interesting mathematical properties. First of all, 666 is equal to the sum of the squares of the first seven primes (seven being considered a magic number, as an extra little fact). In other words:

666 = 2² + 3² + 5² + 7² + 11² + 13² + 17²

As well as this cool coincidence, 666 is the sum of palindromic (meaning it reads the same backwards as it does forwards) cubes.

666 = 1³ + 2³ + 3³ + 4³ + 5³ + 6³ + 5³ + 4³ + 3³ + 2³ + 1³

And just to add to the weirdness, the 6³ in the centre is shorthand for 6 x 6 x 6.

So, is 666 the Number of the Beast, or the Number of the Numerologist?